VLTI Baseline Vector and OPD
(16.08.2007)
1. Initialisations
> restart:
> Digits := 16;
![]()
> deg2rad := evalf(180./Pi);
![]()
> mas2rad := deg2rad*1000.*3600.;
![]()
> with(linalg): # Linear algebra package
Warning, the protected names norm and trace have been redefined and unprotected
> with(inttrans): # Integral transforms
Warning, the name hilbert has been redefined
> latitude := -24.62528; # Latitude of Paranal obs., in degrees (about 24 degrees South, South counts as negative)
![]()
> longitude := -70.40278; # Longitude of Paranal obs., in degrees (about 70 degrees West, West counts as negative)
![]()
2. Computing the number of days since J2000.0 (Jan 1st 2000, 12:00 UT)
> # For the definition of ydays we voluntarily avoid the leap year 2004
> ydays := yy -> piecewise(yy=1999,-366.5,yy=2000,-1.5,yy=2001,364.5,yy=2002,729.5,yy=2003,1094.5);
![]()
> # On leap years add one to the days of the month starting with March
> mdays := mm -> piecewise(mm=1,0,mm=2,31,mm=3,59,mm=4,90,mm=5,120,mm=6,151,mm=7,181,mm=8,212,mm=9,243,mm=10,273,mm=11,304,mm=12,334);
![]()
> yy := 2003: # 2003-01-01
> mm := 01:
> dd := 01:
>
> # In this application we leave UT undetermined for the moment
> # UT := 23.00 + 20/60. + 44.5/3600.; # Universal Time of observation (23h20min44.500 for alfpsa)
>
> days2K := ydays(yy) + mdays(mm) + dd + UT/24.0; # Number of days since J2000.0 (J2000.0 corresponds to 1200 hrs UT on Jan 1st 2000 AD)
![]()
3. Computing the Sun coordinates (right ascension, declination)
> # Computes Sun coordinates
> Ls := 280.461 + 0.9856474 * days2K; # Mean longitude of the sun
![]()
> Ls := Ls - 360.*floor(Ls/360.); # Ls (in degrees) is in the interval [0.,360.]
![]()
> gs := 357.528 + 0.9856003 * days2K; # Mean anomaly of the sun
![]()
> gs := gs - 360.*floor(gs/360.); # gs (in degrees) is in the interval [0.,360.]
![]()
> lambda0 := Ls + 1.915 * sin(gs/deg2rad) + 0.020 * sin(2*gs/deg2rad); # Ecliptic longitude of the Sun, in degrees
![]()
![]()
![]()
> lambda0 := lambda0 - 360.*floor(lambda0/360.);
![]()
![]()
![]()
![]()
> epsilon0 := 23.439 - 0.0000004 * days2K; # Obliquity of the ecliptic plane
![]()
> Ys := cos(epsilon0/deg2rad) * sin(lambda0/deg2rad);
![]()
![]()
![]()
![]()
![]()
> Xs := cos(lambda0/deg2rad);
![]()
![]()
![]()
![]()
> alpha0 := evalf(arctan(Ys,Xs)*deg2rad);
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
> delta0 := deg2rad*arcsin(sin(epsilon0/deg2rad)*sin(lambda0/deg2rad));
![]()
![]()
![]()
![]()
![]()
4. Computing the Local Sidereal Time (LST)
> # Compute Local Sidereal Time (LST)
> LST := 100.46 + 0.985647 * days2K + longitude + 15.0*UT; # LST is expressed in degrees
![]()
> LST := LST-3*360.;
![]()
> LSTS := LST*240.; # LST in seconds, as given in the FITS header
![]()
>
> LSTH := (LST)/15;
![]()
> LSTM := (LSTH-20.)*60;
![]()
> LSTS := (LSTM-36.)*60.; # LST is 00:40:08.0745
![]()
5. Sun altitude and azimuth
> # Coumpute Hour Angle of the Sun, Altitude and Azimuth (HAS, ALTS, AZS)
> HAS := LST - alpha0;
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
> sas := sin(delta0/deg2rad)*sin(latitude/deg2rad)+cos(delta0/deg2rad)*cos(latitude/deg2rad)*cos(HAS/deg2rad);
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
> ALTS := arcsin(sas);
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
> altsdeg := ALTS*deg2rad;
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
> plot(altsdeg,UT=0..24);
![[Maple Plot]](images/maple_fringe_vis_example116.gif)
> # Let's consider night time is from 00:00 UT to 09:00 UT
6. Target altitude and azimuth
> # Compute Hour Angle of the target
> # Target is Sirius
> rah := 06.00: # RA = 6 hours and...
> ram := 45.00: # 45 minutes and ...
> ras := 08.90: # 8.9 seconds
> alpha := rah*15+ram*15/60+ras*15/3600; # Target right ascension, in degrees
![]()
>
> decd := -16: # Declination is -16 degrees and ..
> decm := 42: # 42 minutes and...
> decs := 58.5: # 58.5 seconds
> dd := abs(decd); # we keep the absolute value and sign of the declination
![]()
> ds := sign(decd);
![]()
> delta := ds*(dd+decm/60.+decs/3600.); # Target declination, in degrees
![]()
> HA := LST - alpha; # Hour Angle in degrees
![]()
> HA := HA + 360.;
![]()
> HA/15; # Hour Angle in Hours
![]()
> plot(HA/15,UT=5..9); # Plot hour angle in hours: 24 hours=360 degrees
![[Maple Plot]](images/maple_fringe_vis_example124.gif)
> # Compute Altitude and Azimuth (ALT, AZ) of the target
> sa := sin(delta/deg2rad)*sin(latitude/deg2rad)+cos(delta/deg2rad)*cos(latitude/deg2rad)*cos(HA/deg2rad);
![]()
> altitude := arcsin(sa);
![]()
> altdeg := altitude*deg2rad; # Force floating point evaluation of altitude, convert to degrees
![]()
> plot(altdeg,UT=0..24);
![[Maple Plot]](images/maple_fringe_vis_example128.gif)
> # During the night time (00:00 UT to 09:00 UT), the target is at reasonable elevation (above 20 degrees) only until 07:00 UT. We will later on restrict our time exploration to the range 00:00 - 07:00 UT.
> tazx := cos(delta/deg2rad)*sin(HA/deg2rad);
![]()
> tazy := sin(latitude/deg2rad)*cos(delta/deg2rad)*cos(HA/deg2rad)-cos(latitude/deg2rad)*sin(delta/deg2rad);
![]()
> azimuth := -arctan(tazy,tazx); # -atan2(tazy,tazx);
![]()
> evalf(arctan(-1,1)*deg2rad);
![]()
> azd := azimuth*deg2rad;
![]()
> azdeg := piecewise(azd<0,azd+360,azd>=0,azd);
![]()
![]()
![]()
![]()
> plot(azdeg,UT=0..7);
![[Maple Plot]](images/maple_fringe_vis_example138.gif)
> plot(270-azdeg,UT=0..10); # VisCalc azimuth plot
![[Maple Plot]](images/maple_fringe_vis_example139.gif)
8. Compute baseline vector.
> nu := -18.984:
> psi := evalf(nu/deg2rad); # Rotation of the platform (can be set to nu=-18.984 degrees as above)
![]()
> R := matrix(3,3,[[cos(psi),sin(psi),0],[-sin(psi),cos(psi),0],[0,0,1]]);
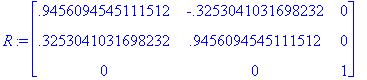
> # Exact coordinates for the stations are U1 -16.000 -16.000 -9.925 -20.335, U3 64.0013 47.9725 44.915 66.183
> ntel1 := vector([-16,-16,0]); # Nominal U,V position for station UT1
![]()
> ntel2 := vector([64.0013,47.9725,0]); # Nominal U,V position for station UT3
![]()
> rtel1 := evalm(R &* ntel1); # N-E coordinates of station E0, as given in the FITS header
![]()
> rtel2 := evalm(R &* ntel2); # N-E coordinates of station G0, as given in the FITS header
![]()
>
>
> BN := rtel2[2] - rtel1[2]; # N-S ground projection of the baseline vector
![]()
> BE := rtel2[1] - rtel1[1]; # E-W ground projection of the baseline vector
![]()
> BA := rtel2[3] - rtel1[3]; # Elevation difference between telescope 1 and 2
![]()
> Bg:= vector([BN,BE,BA]);
![]()
> harad := HA/deg2rad;
![]()
> decrad := delta/deg2rad;
![]()
> decrad := -.2917536010865021;
![]()
> #harad := -15./60./deg2rad; # Overwrite, H = 1 min
> #decrad := 0./deg2rad; # Overwrite
> lat := latitude/deg2rad;
![]()
> L := matrix(3,3,[[-sin(lat),0,cos(lat)],[0,-1,0],[cos(lat),0,sin(lat)]]); # Belle, Eq. 4.108, p. 44
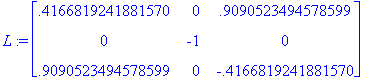
> B := evalm(L &* Bg);
![]()
> T := matrix(3,3,[[sin(harad),-cos(harad),0],[-sin(decrad)*cos(harad),-sin(decrad)*sin(harad),cos(decrad)],[cos(decrad)*cos(harad),cos(decrad)*sin(harad),sin(decrad)]]);
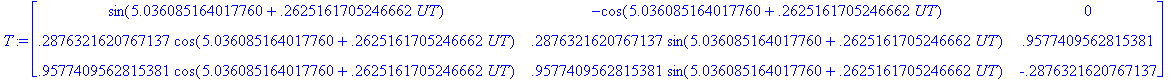
> UVW := evalm(T &* B);
![]()
![]()
![]()
> u := UVW[1];
![]()
> plot(u,UT=0..9);
![[Maple Plot]](images/maple_fringe_vis_example161.gif)
> v:= UVW[2];
![]()
> plot(v,UT=0..9);
![[Maple Plot]](images/maple_fringe_vis_example163.gif)
> plot([[u,v,UT=0..9],[-u,-v,UT=0..9]],-100..100,-100..100);
![[Maple Plot]](images/maple_fringe_vis_example164.gif)
>
> w := UVW[3];
![]()
> plot(w,UT=3..3.5);
![[Maple Plot]](images/maple_fringe_vis_example166.gif)
> rho := sqrt(u*u+v*v);
![]()
![]()
> #plot(rho,decrad=-30/deg2rad..-20/deg2rad);
9. Model Uniform Disk
> lambda := 2.2e-6; # Central wavelength in meters (1 micron = 1e-6 meter)
![]()
> theta := 6.00/mas2rad; # 6 mas, converted to radian
![]()
> rho := sqrt(u*u + v*v);
![]()
![]()
> C(rho);
![]()
![]()
> plot(rho,UT=3..3.5);
![[Maple Plot]](images/maple_fringe_vis_example175.gif)
> uda := Pi*theta*abs(rho)/lambda;
![]()
![]()
> evalf(uda);
![]()
![]()
> udvs := 2*BesselJ(1,uda)/uda;
![]()
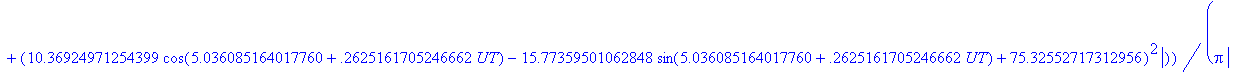
![]()
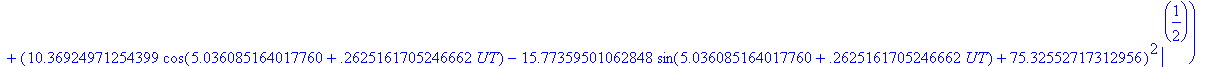
> udv := evalf(abs(udvs));
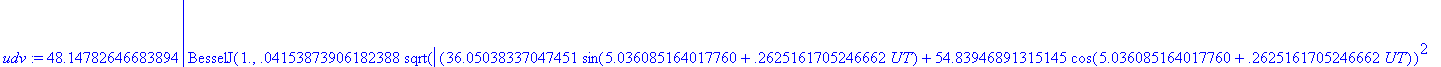
![]()
![]()
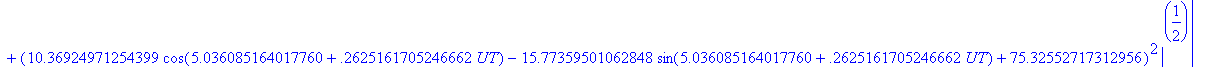
> plot(udv,UT=0..10); # Visibility values
![[Maple Plot]](images/maple_fringe_vis_example188.gif)
> # Phase
> # if (udvs < 0.0) phase = pi; else if (udvs >= 0.0) phase = 0.0;
9. Model Gaussian
> lambda := 2.20e-6; # Central wavelength in meters (1 micron = 1e-6 meter)
![]()
> uCoord := u/lambda;
![]()
> vCoord := v/lambda;
![]()
> rho := sqrt(uCoord*uCoord + vCoord*vCoord);
![]()
![]()
![]()
![]()
> plot(evalf(rho),UT=0..1); # Baseline plot
![[Maple Plot]](images/maple_fringe_vis_example196.gif)
>
> gaussFwhm := 6.00/mas2rad; # 6 mas, converted to radian
![]()
> udv := exp( (-2 * Pi * Pi * gaussFwhm * gaussFwhm * rho * rho)/(8 * log(2)) );
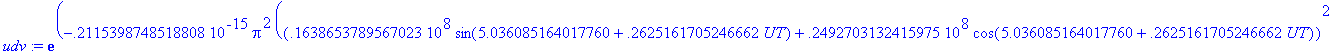
![]()
> plot(evalf(udv),UT=0..10,0..0.025); # Visibility values
![[Maple Plot]](images/maple_fringe_vis_example200.gif)
> phase := 0; # for a Gaussian the phase is always zero
![]()
10. Model Binary Disc
> lambda := 2.2e-6; # Central wavelength in meters (1 micron = 1e-6 meter)
![]()
> uCoord := u/lambda;
![]()
> vCoord := v/lambda;
![]()
> rho := sqrt(uCoord*uCoord + vCoord*vCoord);
![]()
![]()
> brightRatio := 1.0; # Brightness ratio
![]()
> r1 := 4.0/2/mas2rad; # Radius 1 (half-diameter), converted to radian
![]()
> r2 := 3.0/2/mas2rad; # Radius 2 (half-diameter), converted to radian
![]()
> theta := 37; # Position angle, converted to radian
![]()
> sep := 10/mas2rad; # Separation 12 mas, converted to radian
![]()
> ud1 := 2.0*Pi*rho*r1;
![]()
![]()
> ud2 := 2.0*Pi*rho*r2;
![]()
![]()
> bessel1 := BesselJ(1,ud1);
![]()
![]()
> bessel2 := BesselJ(1,ud2);
![]()
![]()
> udv_den := ( Pi*Pi* (brightRatio*brightRatio*r1*r1*r1*r1*uCoord*uCoord + brightRatio*brightRatio*r1*r1*r1*r1*vCoord*vCoord + 2*brightRatio*r1*r1*r2*r2*uCoord*uCoord + 2*brightRatio*r1*r1*r2*r2*vCoord*vCoord + r2*r2*r2*r2*uCoord*uCoord + r2*r2*r2*r2*vCoord*vCoord ));
![]()
![]()
> udv_num := 2*r2*bessel2*brightRatio*r1*bessel1*cos(2*Pi*sep*(cos(theta)*uCoord + sin(theta)*vCoord)) + brightRatio*brightRatio*r1*r1*bessel1*bessel1 + r2*r2*bessel2*bessel2;
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
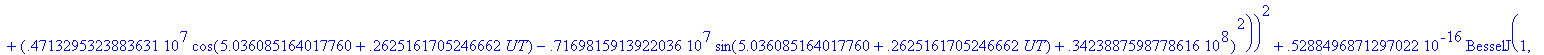
![]()
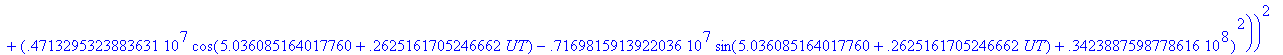
>
> udv2 := udv_num / udv_den;

![]()
![]()
![]()
![]()
![]()
![]()
![]()
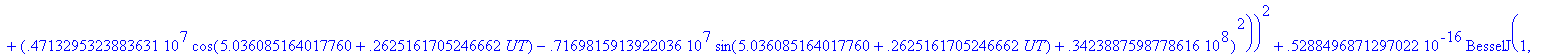
![]()
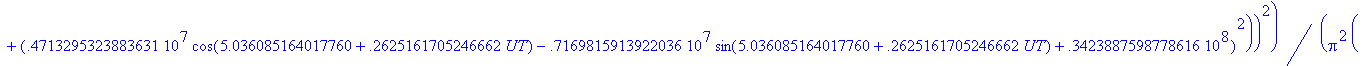
![]()
![]()
> udv := sqrt(udv2);

![]()
![]()
![]()
![]()
![]()
![]()
![]()
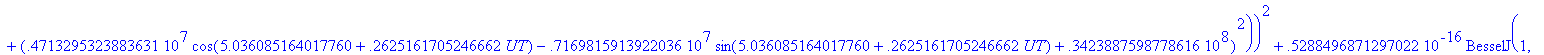
![]()
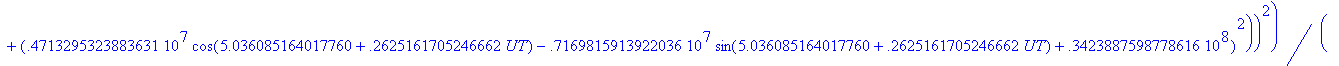
![]()
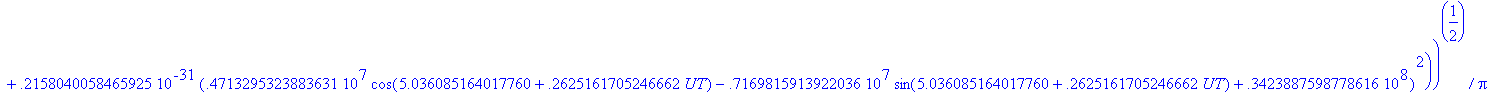
> plot(evalf(udv),UT=0..10); # Visibility values
![[Maple Plot]](images/maple_fringe_vis_example259.gif)
> I1 := brightRatio*r1*bessel1;
![]()
![]()
> I2 := r2*bessel2;
![]()
![]()
> u := cos(theta)*uCoord + sin(theta)*vCoord;
![]()
![]()
> nominatorPhase := I1*sin(Pi*u*sep) - I2*sin(Pi*u*sep);
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
> denominatorPhase := I1*cos(Pi*u*sep) + I2*cos(Pi*u*sep);
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
> phase := arctan(nominatorPhase/denominatorPhase);
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
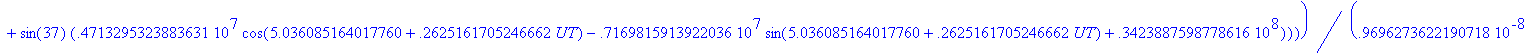
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
> plot(evalf(phase), UT=0..10);
![[Maple Plot]](images/maple_fringe_vis_example301.gif)
>
>
>
>
>
>
>
>
>
>
>
>
>
>
>